MATH 481, Project 4: Population Models

Malthus' model

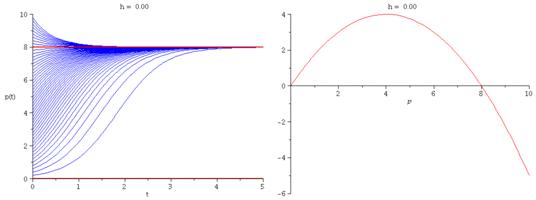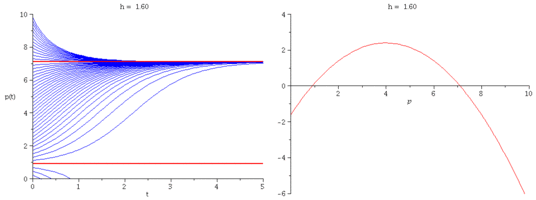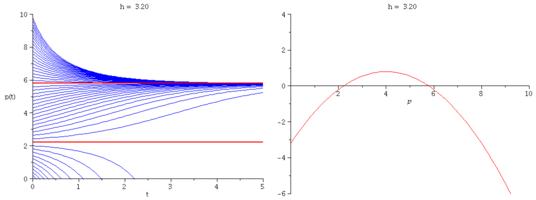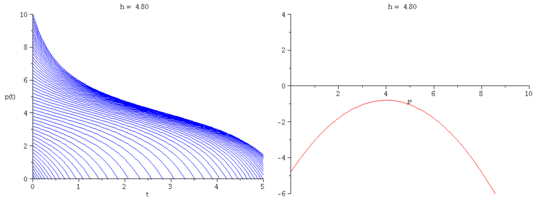
We modify Verhulst's model by introducing a constant harvesting rate $h$: \[ \frac{dp}{dt} = r\Big(1-\frac{p}{k}\Big)p - h. \]

What is the critical harvesting value $h_c$ so that the population has no chance of survival when $h > h_c$? Express $h_c$ in terms of $r$ and $k$.
Plot representative solution curves for several values of $h$.
This animation can give you ideas for your article:

Explain the logic behind the derivation of the predator-prey model \begin{align*} \frac{dx}{dt} &= ( a_1 - b_{11} x - b_{12} y)x, \\ \frac{dy}{dt} &= (-a_2 + b_{21} x - b_{22} y)y. \end{align*}
Describe the essential features of the system's phase portrait and its equilibria. Qualitatively, there are two distinct cases, depending on whether $a_1/b_{11} < a_2/b_{21}$ or $a_1/b_{11} > a_2/b_{21}$. Explain what that is so, and show a sample phase portrait for each case.

Add bibliographic items (in your *.bib file) as needed. Here are a couple of items to get you started.

Thomas Robert Malthus. Essay on the Principle of Population as it affects the future improvement of society. With Remarks on the Speculations of Mr. Goodwin, M. Condorcet and other writers. 1st ed. (Printed anonymously and ascribed to J. Johnson in St. Paul’s Church-yard). London, 1798.
Pierre-François Verhulst, Notice sur la loi que la population suit dans son accroissement, Correspondance Mathématique et Physique, 10, 1838, 113–121.